![]()
Application of stationary ‘ throwbox’ or message-buffers in DTN enhances the rate of contacts between the mobile agents and consequently reduces their inter contact delay. The buffer-time, i.e., the time for which a message-copy resides in a particular buffer, happens to be a very crucial issue as different types of overheads – such as number of redundant message copies, CPU consumptions, buffer space - are directly related to it. On the other hand, from the perspective of an information dissemination process, higher buffer-time is helps to increase the achieved coverage, i.e., the number of distinct nodes which could receive a given piece of information. Therefore, it is essential to understand the optimal buffer time to achieve a certain coverage in an information dissemination process in DTN under a given cost. However, the analysis of coverage under the constraint of buffer time using traditional mathematical tools such as mean filed theory, epidemic dynamics etc happens to be an extremely difficult task. In this work we employed a novel strategy to analyze this coverage. Specifically, we have shown that a DTN can be mathematically modeled as a bipartite network and therefore various question related to DTN can be answered by converting them appropriately for the corresponding bipartite network. In this work, we primarily focused on the analysis of the information dissemination process in DTN through this novel strategy. The details of the work can be found in Ref [1].
Initial Results
1. Through extensive simulation and theoretical work, it has been identified and established that the information dissemination process in DTN can be visualized as the evolution of bipartite network and therefore, the coverage of the information dissemination process can be analyzed by computing the largest component size in the one mode projection of the bipartite network after application of a suitable threshold (see Figure 1).
2. In addition, it has also been identified that
a. Arbitrarily increasing the number of agents participating in the dissemination process in DTN cannot increase the coverage once the system has reached the stationary state for a given buffer time.
b. The following equation shows the relationship between the achieved coverage (i.e., Gd which is equal to the largest component size of the thresholded one-mode projection of the corresponding bipartite network i.e. Gb), number of places (N), average social participation of the agents (µ) and employed buffer time (b) in DTN. It can be seen from the equation that Gd varies inversely with N2 and directly with µ2 (A, C and α are constants).
![]()
c. It is possible to design an optimal buffer time for a desired cost of coverage.
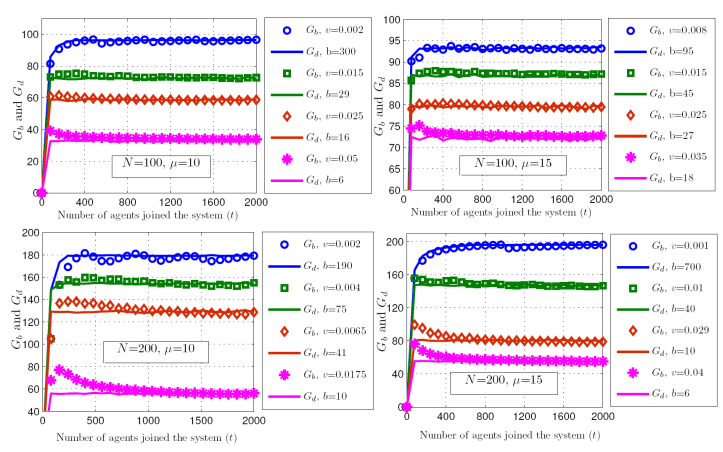
Figure 1: Comparison of the achieved coverage (Gd) in the information dissemination process in DTN and the size of the largest component in the thresholded one mode projection of the corresponding bipartite graph (Gb). The notations - b and v denote the buffer time in DTN and a time varying threshold in bipartite network respectively.
Future Plans
1. It has been assumed that the agents arrive in a sequential fashion. However, to make it realistic, we need to consider the overlapping life spans of the agents.
2. It has been also assumed that the agents select the next place to be visited in a fully preferential fashion. However, in reality, the selection of the next place incurs a little bit randomness. Therefore, the next goal is to test the whole framework incorporating the randomness.
Publications
[1] Information dissemination dynamics in delay tolerant network : a bipartite network approach, Sudipta Saha, Niloy Ganguly and Animesh Mukherjee, In proceedings of ACM MobiOpp 2012, Zurich, Switzerland.